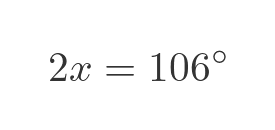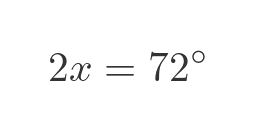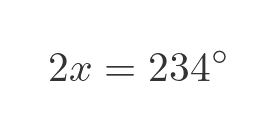Angle at the centre of a circle is twice the angle at the circumference

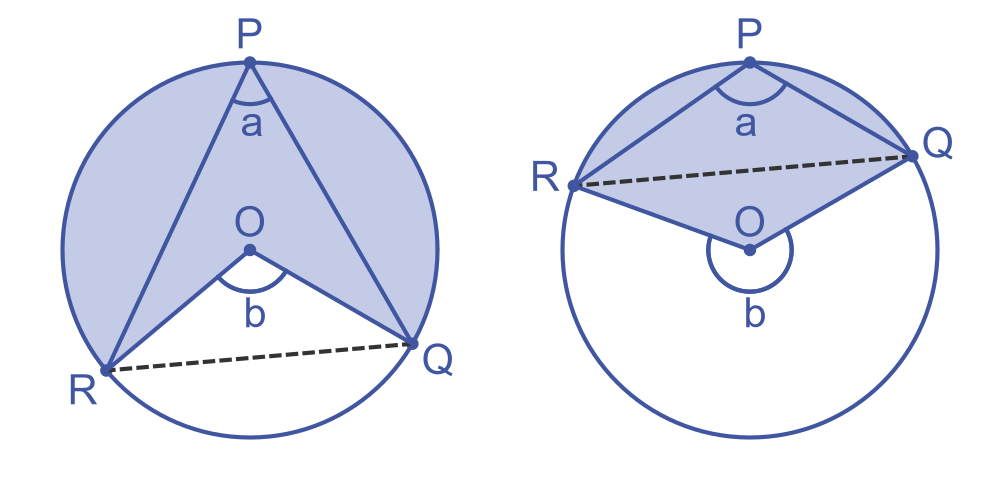
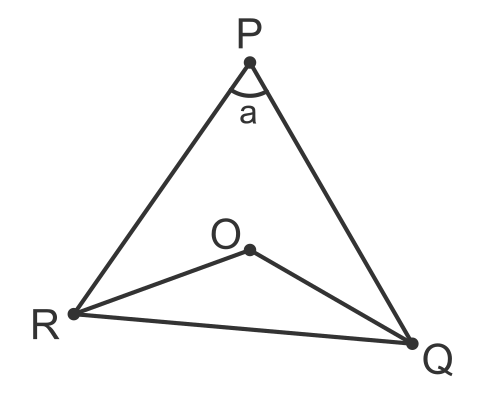
For any chord QR, we can draw two lines to form an angle at the centre of the circle, marked as angle b in the diagram below. We can also draw two lines to some point P on the circumference to form an angle at the circumference, marked as angle a.

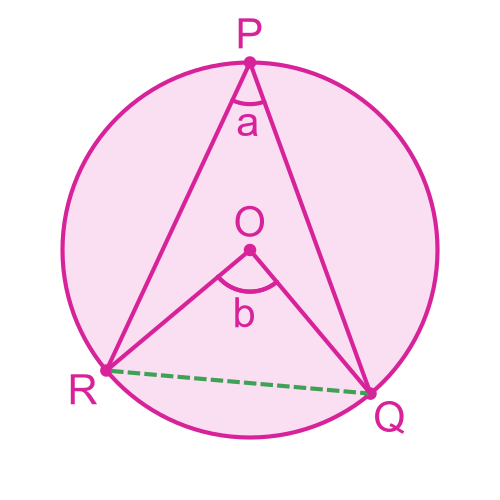
The angle at the centre of a circle theorem states that the angle at the centre will always be twice the angle at the circumference. In other words angle b will always be twice angle a.

Video

Here is a video on this topic:

Alternative shapes

If we move the points P, Q and R around the circumference, the diagram looks different. We need to be able to recognise these different forms and understand how to apply the theorem in each case. Here are a couple of examples:

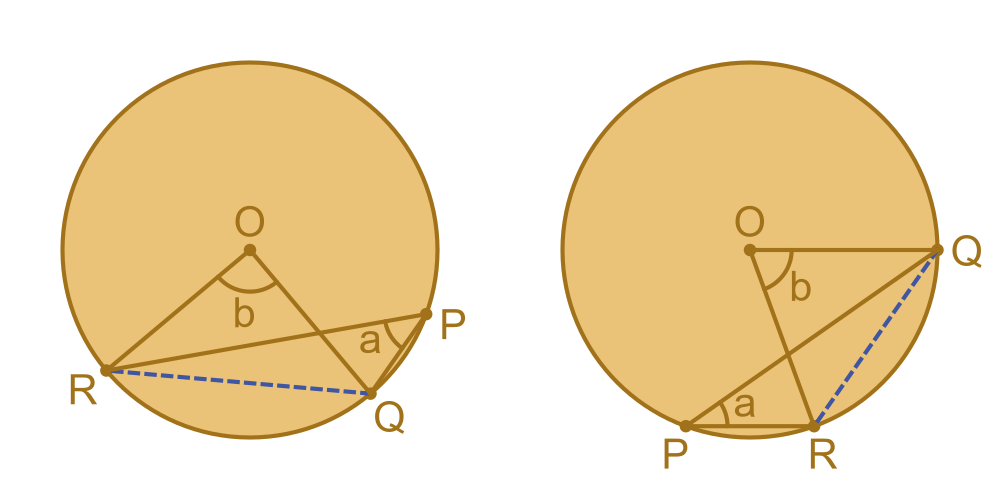
On the left, due to the position of P, the line PR crosses the line OQ. It is important to recognise that this is still the same situation, even though it looks a little different. The chord RQ still forms an angle b at the centre, and an angle a at the circumference, and it is still true that angle b is twice angle a.

On the right of the diagram, the line PQ crosses the line OR, but it is still an example of the same theorem.

Here is another slightly different arrangement which we need to recognise:

This time the point P is in the minor sector formed by the lines OR and OQ.

As we saw earlier, angle b is always in the opposite sector to the angle a. When angle a is in the minor sector, it will be an obtuse angle (that is, an angle that is greater than 90° but less than 180°). Since b is twice a, it will be a reflex angle (that is, an angle that is greater than 180°). Again, don't be confused by the different appearance of the diagram.

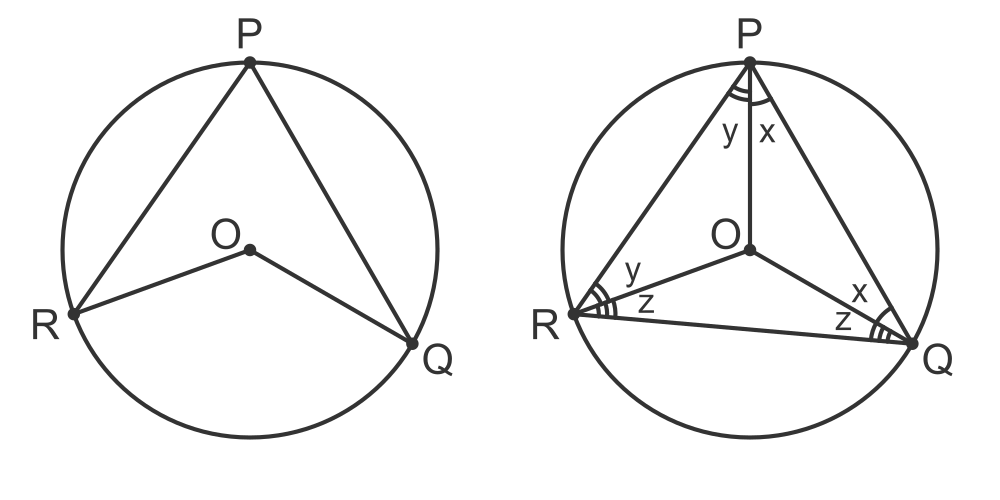
To illustrate this, here are two typical cases, with the sector containing a shaded, and the sector containing b left white:

The circle on the left shows a in the major sector, so a is acute and b is obtuse. The circle on the right shows a in the minor sector, so a is obtuse and b is reflex.

Proof

To prove this theorem, we start by adding two extra lines, OP and RQ (shown on the left of this diagram):

Notice that OP, OQ and OR are all radii of the circle. We know that 2 radii form an isosceles triangle.

This means that:

• Triangle PQO is an isosceles triangle. The angles at the base of an isosceles triangle are equal. We will call the angle x.
• Similarly, the two angles at the base of triangle PRO are equal, and we will call the angle y.
• And the two angles at the base of triangle QRO are equal, and we will call the angle z.

We can find the three angles of the triangle PQR, from the diagram:

• Angle QPR is x + y.
• Angle PQR is x + z.
• Angle PRQ is y + z.

Since we know that the three angles in a triangle add up to 180°, this gives us:

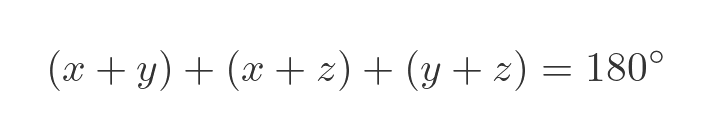
This can be rearranged as:

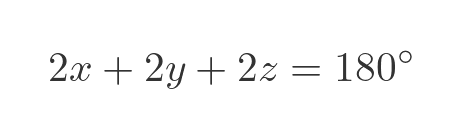
Here is the triangle PQR redrawn to show the angle a:

We can see that the angle at a is equal to x + y from the previous diagram. This means that 2a is equal to 2x + 2y.

We can replace 2x + 2y with 2a in the earlier equation, to get:

Which means:

Now let's look at the triangle QRO:

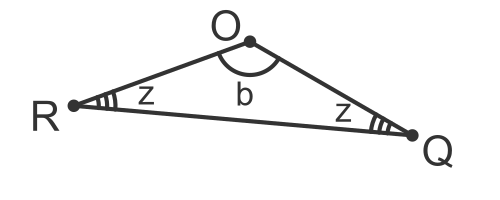
For the earlier diagrams, we know that the angle at O is b, and the angles at Q and R are both equal to z. Since the angles in the triangle add up to 180° we can see that:

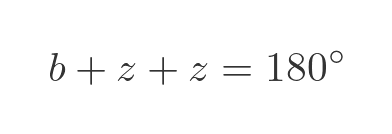
We can solve for b:

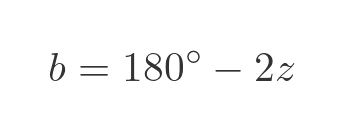
Compare this with the previous value for 2a:

This proves that angle b is twice angle a.

Questions

Here are some exam-style questions on the angle at the centre of a circle theorem. You can click the button at the end of each question to reveal the answer.

The Circle Theorems Workbook contains additional questions and answers related to circle theorems.

Question 1

In the diagram, A, B and C are three points on the circumference of a circle with centre O. Angle BOC has a value of 106°.

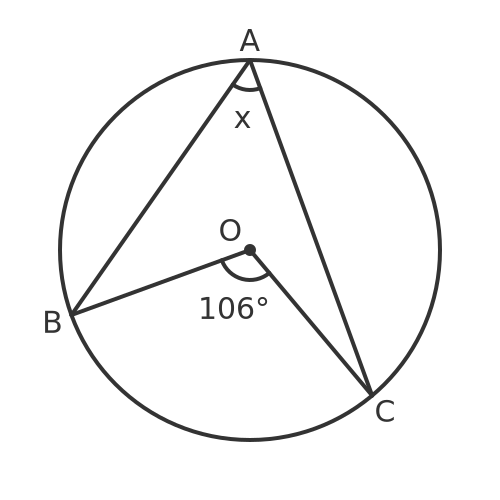
What is the value of the angle x? Give a reason for your answer.

Show answer 1

Question 2

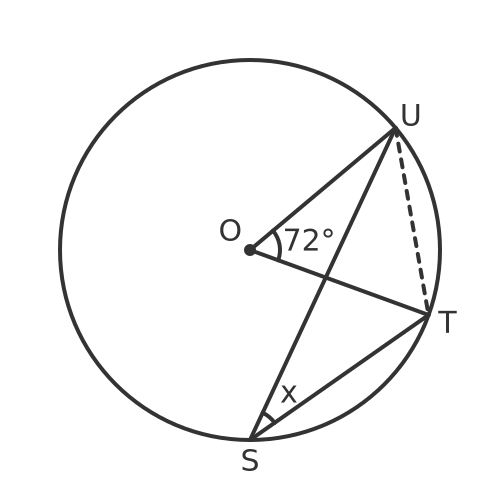
The diagram shows three points S, T and U on the circumference of a circle with centre O. Angle TOU has a value of 72°.

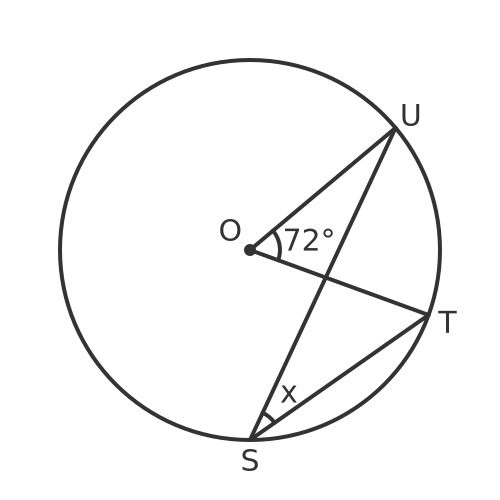
What is the value of the angle x? Show your working.

Show answer 2

Question 3

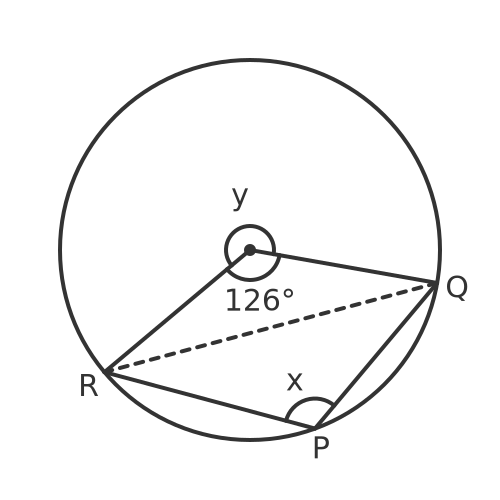
The diagram shows three points P, Q and R on the circumference of a circle with centre O. Angle ROQ has a value of 126°.

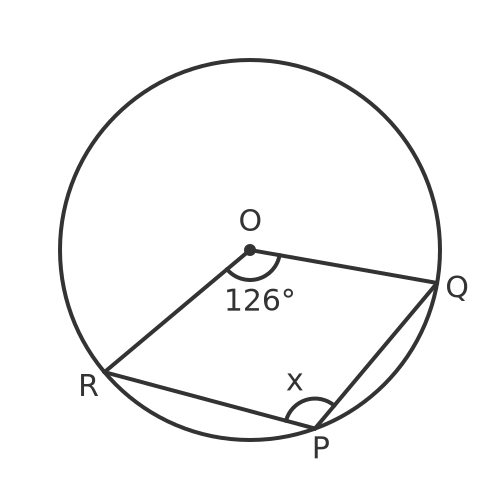
What is the value of the angle x? Show your working.

Show answer 3

Question 4

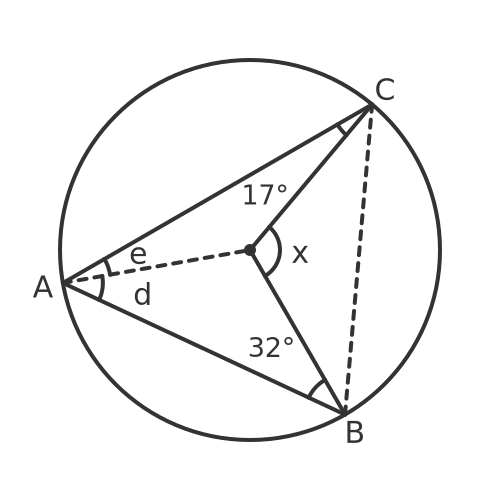
In the diagram, A, B and C are three points on the circumference of a circle with centre O. Angle ACO has a value of 17°, and ABO has a value of 32°.

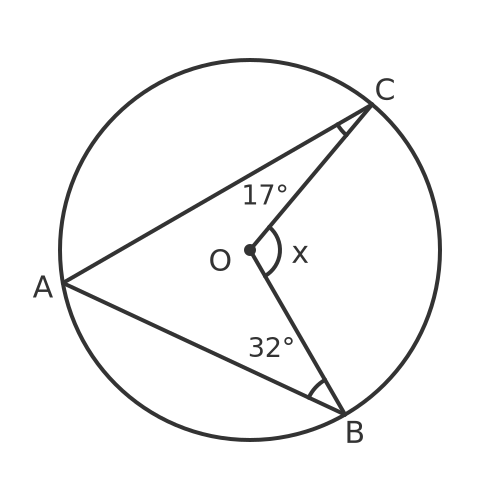
What is the value of the angle x? Explain your answer

Show answer 4

See also

• Parts of a circle
• Perpendicular bisector of chord theorem
• Angle in a semicircle is 90°
• Angles in the same segment of a circle are equal
• Opposite angles in a cyclic quadrilateral add up to 180°
• Tangent and radius of a circle meet at 90°
• Two tangents from a point have equal length
• Alternate segment theorem
• Two radii form an isosceles triangle